
[Home] [Front Handspring Vault] [Iron Cross] [Handstand]


Internal Muscular Force Muscular strength is considered to be the ability to do work against resistance or the capacity to exert force Arnheim and Prentice, 1993) and is derived through muscle tension produced during muscle contraction. The outcome is a force internal to the human system. This internal force produces tension on the skeletal system, resulting in angular motion about a joint. Examples of this phenomenon are shown in Figures 2-4 by furnishing illustrations of gymnastics movements and associated muscle forces.
Resolution of force
Resolution of force or vector resolution is an example of a biomechanical technique that provides a means of establishing the two component forces directly associated with a net force. This net force can be responsible for a movement and is known as the resultant force (Figure 1). An explanation is now possible for why a particular force results in a specific movement when the resultant vector is known. This leads to resolving the component forces. Component forces provide a clearer picture of what the resultant force contributes to the performance.
Component forces
© 1997 USA Gymnastics. All rights reserved.
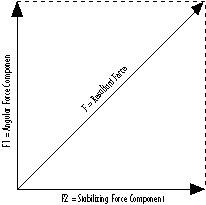 Angular and stabilizing vectors represent the
two components derived from a resultant force. Figure 1 illustrates the
relationship between the resultant vector and component forces. When dealing
with internal forces created from muscle contractions, the resultant force (F)
is applied at the muscle insertion located on the attachment (apophysis) of the
bone to be mobilized and is directed toward the muscle origin. This resultant
force is directed through the belly of the particular muscle. The angular vector
is directed perpendicular to the bone that is being moved and the stabilizing
vector is directed along the same bone, into the joint (Kreighbaum and Barthels,
1996). Consequently, the angular force component is responsible for moving the
skeletal part, while the stabilizing force component serves as a means of adding
to joint integrity (stability). The angular vector (F1) and stabilizing vector
(F2) begin from the point of force application and are directed at right angles,
one to the other. Figures 2-4 provide examples of resultant vectors and their
component vectors, originating from concentric muscle contractions during the
performance of gymnastics skills.
Angular and stabilizing vectors represent the
two components derived from a resultant force. Figure 1 illustrates the
relationship between the resultant vector and component forces. When dealing
with internal forces created from muscle contractions, the resultant force (F)
is applied at the muscle insertion located on the attachment (apophysis) of the
bone to be mobilized and is directed toward the muscle origin. This resultant
force is directed through the belly of the particular muscle. The angular vector
is directed perpendicular to the bone that is being moved and the stabilizing
vector is directed along the same bone, into the joint (Kreighbaum and Barthels,
1996). Consequently, the angular force component is responsible for moving the
skeletal part, while the stabilizing force component serves as a means of adding
to joint integrity (stability). The angular vector (F1) and stabilizing vector
(F2) begin from the point of force application and are directed at right angles,
one to the other. Figures 2-4 provide examples of resultant vectors and their
component vectors, originating from concentric muscle contractions during the
performance of gymnastics skills.